Give me the ncert class 10 solution of chapter ares related to circles .Find the best and easiest way t0 solve this question . Sir please help me to find the exercise 12.3 question no. 10 . The area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region. (Use π = 3.14 and √3 = 1.73205).
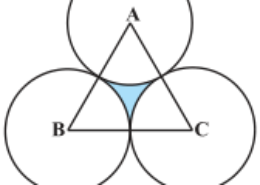
ABC is an equilateral triangle.
∴ ∠ A = ∠ B = ∠ C = 60°
There are three sectors each making 60°.
Area of ΔABC = 17320.5 cm2
⇒ √3/4 ×(side)2 = 17320.5
⇒ (side)2 =17320.5×4/1.73205
⇒ (side)2 = 4×104
⇒ side = 200 cm
Radius of the circles = 200/2 cm = 100 cm
Area of the sector = (60°/360°)×π r2 cm2
= 1/6×3.14×(100)2 cm2
= 15700/3cm2
Area of 3 sectors = 3×15700/3 = 15700 cm2
Thus, area of the shaded region = Area of equilateral triangle ABC – Area of 3 sectors
= 17320.5-15700 cm2 = 1620.5 cm2