How i solve this problem of class 10th of ncert math of exercise 6.6 of question no5(2). What is the best way to solve this problem because it is also an important question In Figure, AD is a median of a triangle ABC and AM ⊥ BC. Prove that : (ii) AB2 = AD2 – BC.DM + 2 (BC/2) 2
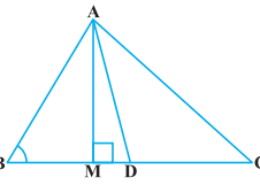
By applying Pythagoras Theorem in ∆ABM, we get;
AB2 = AM2 + MB2
= (AD2 − DM2) + MB2
= (AD2 − DM2) + (BD − MD) 2
= AD2 − DM2 + BD2 + MD2 − 2BD × MD
= AD2 + BD2 − 2BD × MD
= AD2 + (BC/2)2 – 2(BC/2) MD
= AD2 + (BC/2)2 – BC MD
Hence, proved.