Home/ Questions/Q 11871
In Process
Adv
AnilSinghBora
  • 0
Guru
Asked: 2021-08-12T15:43:11+05:30 In:

In Fig.9.33, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that:(iii) ar (ABC) = 2 ar (BEC) Q.5(3)

  • 0
In Fig.9.33, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that:(iii) ar (ABC) = 2 ar (BEC) Q.5(3)

Please give me the best way for solving the problem of class 9th ncert math of Areas of Parallelograms and Triangles chapter of math of class 9th of exercise 9.4 of question no 5(3) what is the tricky way for solving this question In Fig.9.33, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that:(iii) ar (ABC) = 2 ar (BEC)

area of parallelograms and triangles ncertbest math ncert solutionexercise 9.4 ncertncert class 9th solution
Share

    1 Answer

    1. Guru

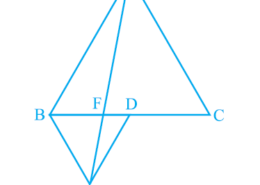
      ar(ΔABE) = ar(ΔBEC) [Common base BE and BE || AC]

      ar(ΔABF) + ar(ΔBEF) = ar(ΔBEC)

      From eqn (i), we get,

      ar(ΔABF) + ar(ΔAFD) = ar(ΔBEC)

      ar(ΔABD) = ar(ΔBEC)

      ½ ar(ΔABC) = ar(ΔBEC)

      ar(ΔABC) = 2 ar(ΔBEC)

      Hence proved

      • 0
    Leave an answer

    Leave an answer

    Browse

    Choose from here the video type.

    Put Video ID here: https://www.youtube.com/watch?v=sdUUx5FdySs Ex: "sdUUx5FdySs".

    Captcha Click on image to update the captcha.

    Related Questions

    Adv