In ncert class 10 chapter areas related to circle what is the best solution of the exercise 12.3 question number 5 ,Please help me to solve this question in a easiest and simplest way . Give me the best solution of this question .From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in Fig. 12.23. Find the area of the remaining portion of the square.
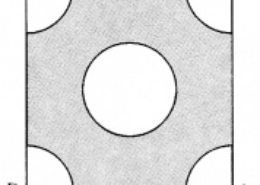
Side of the square = 4 cm
Radius of the circle = 1 cm
Four quadrant of a circle are cut from corner and one circle of radius are cut from middle.
Area of square = (side)2= 42 = 16 cm2
Area of the quadrant = (πR2)/4 cm2 = (22/7)×(12)/4 = 11/14 cm2
∴ Total area of the 4 quadrants = 4 ×(11/14) cm2 = 22/7 cm2
Area of the circle = πR2 cm2 = (22/7×12) = 22/7 cm2
Area of the shaded region = Area of square – (Area of the 4 quadrants + Area of the circle)
= 16 cm2-(22/7) cm2+(22/7) cm2
= 68/7 cm2