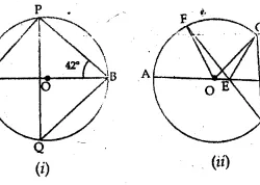
Solution:

In ∆APB,

∠APB = 90° (Angle in a semi-circle)

But ∠A + ∠APB + ∠ABP = 180° (Angles of a triangle)

∠A + 90° + 42°= 180°

∠A + 132° = 180°

⇒ ∠A = 180° – 132° = 48°

But
∠A = ∠PQB

(Angles in the same segment of a circle)

∠PQB = 48^o

(b) (i) in ∆EDC,

(Ext, angle of a triangle is equal to the sum

of its interior opposite angels)

(ii) arc CF subtends ∠COF at the centre and

∠CDF at the remaining part of the circle

∠COF = 2 ∠CDF = 2 ∠CDE

=2 × 32^o = 2 ∠CDE

= 2 × 32^o = 64^o