This is circle based question from Chapter name- circles
Topic – Angle properties of circles
Chapter number- 15
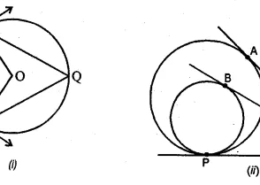
In first part of question it is given that, PA and PB are tangents at a points A and B respectively of a circle with centre O. Q and R are points on the circle.
If ∠APB = 70°, now we have to find (i) ∠AOB (ii) ∠AQB (iii) ∠ARB
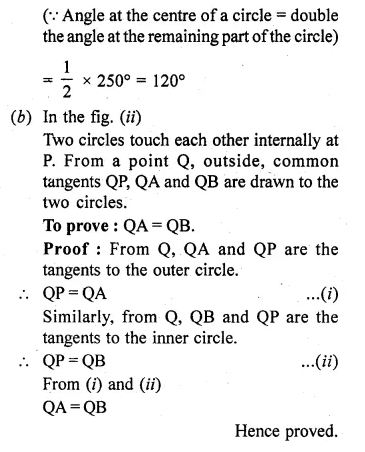
In second part , given that two circles touch internally at P from an external point Q on the common tangent at P, two tangents QA and QB are drawn to the two circles.
Now we have to prove that QA = QB.
ICSE Avichal publication
Understanding ICSE Mathematics
Question no 18




Solution:
(a) To find : (i) ∠AOB, (ii) ∠AQB, (iii) ∠ARB
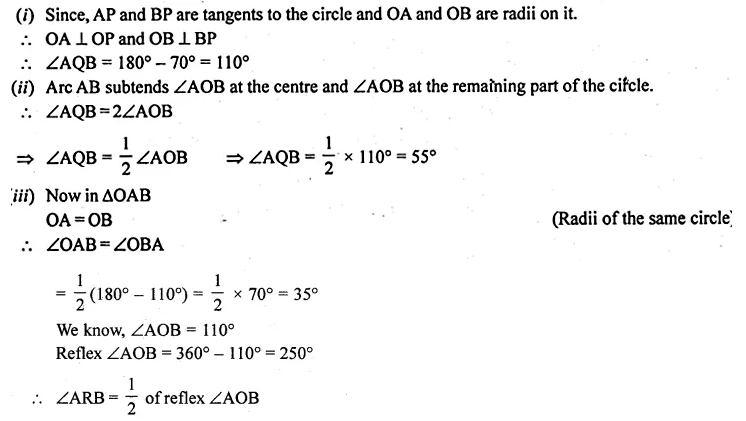
Given: PA and PB are tangents at the points A and B respectively
of a circle with centre O and OA and OB are radii on it.
∠APB = 70°
Construction: Join AB
Num b