This is the basic and conceptual question from Chapter name- circles
Topic – Angle properties of circles
Chapter number- 15
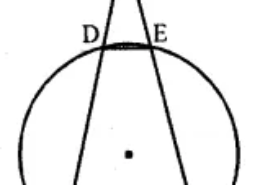
In the given that ABC is an isosceles triangle in which AB = AC and circle passing through B and C intersects sides AB and AC at points D and E
We have to prove that DE || BC.
ICSE Avichal publication
Understanding ICSE Mathematics
Question 14




Solution:
In the given figure,
∆ABC is an isosceles triangle in which AB = AC.
A circle passing through B and C intersects
sides AB and AC at D and E.
To prove: DE || BC
Construction : Join DE.
∵ AB = AC
∠B = ∠C (angles opposite to equal sides)
But BCED is a cyclic quadrilateral
Ext. ∠ADE = ∠C
= ∠B (∵ ∠C = ∠B)
But these are corresponding angles
DE || BC
Hence proved.