This is an important and exam oriented question from Chapter name- circles
Topic – Angle properties of circles
Chapter number- 15
This ques has been asked in 2008 question paper
In the figure we have AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°
Niw we have to Find:
(i) ∠CAD (ii) ∠CBD (iii) ∠ADC
ICSE Avichal publication
Understanding ICSE Mathematics
Question 3(b)



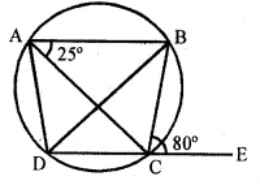

(b) in the figure, AB ∥DC
∠BCE = 80o and ∠BAC = 25o
ABCD is a cyclic Quadrilateral and DC is
Production to E
(i) Ext, ∠BCE = interior ∠A
80o = ∠BAC + ∠CAD
80o = 25o + ∠CAD
∠CAD = 80o – 25o = 55o
(ii) But ∠CAD = ∠CBD
(Alternate angels)
∠CBD = 55o
(iii) ∠BAC = ∠BDC
(Angles in the same segments)
∠BDC = 25o
(∠BAC = 25o)
Now AB ∥ DC and BD is the transversal
∠BDC = ∠ABD
∠ABD = 25o
∠ABC = ∠ABD + ∠CBD = 25o + 55o = 80o
But ∠ABC + ∠ADC = 180o
(opposite angles of a cyclic quadrilateral)
80o + ∠ADC = 180o
∠ADC = 180o – 80o = 100o