Give me the best and simple way for solving the question of class 9th of exercise 9.4 of Areas of parallelograms and triangles chapter of math of question no.8(7) how i solve this question in easy and simple way because it is very important type of question In Fig. 9.34, ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment AX ^ DE meets BC at Y. Show that:(vii) ar(BCED) = ar(ABMN)+ar(ACFG)
AnilSinghBoraGuru
In Fig. 9.34, ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment AX ^ DE meets BC at Y. Show that:(vii) ar(BCED) = ar(ABMN)+ar(ACFG) Q.8(7)
Share



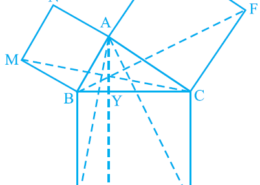

From the figure, we can observe that
ar (∆CED) = ar (∆YXD)+ar (CYXE)
∴ar (∆CED) = ar (ABMN)+ar (ACFG) [From equations (iii) and (vii)].