Yesterday i was doing the question from class 9th ncert book of math of Lines and Angles chapter of exercise 6.1 What is the easiest way for solving it because i was not able to do this question please help me for solving this question of no.5 In Fig. 6.17, POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ROS = ½ (QOS – POS)
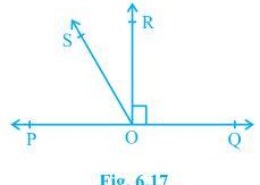
the question, it is given that (OR ⊥ PQ) and POQ = 180°
So, POS+ROS+ROQ = 180°
Now, POS+ROS = 180°- 90° (Since POR = ROQ = 90°)
∴ POS + ROS = 90°
Now, QOS = ROQ+ROS
It is given that ROQ = 90°,
∴ QOS = 90° +ROS
Or, QOS – ROS = 90°
As POS + ROS = 90° and QOS – ROS = 90°, we get
POS + ROS = QOS – ROS
2 ROS + POS = QOS
Or, ROS = ½ (QOS – POS) (Hence proved).