Home/ Questions/Q 11620
In Process
Adv
Sonu
  • 0
Newbie
Asked: 2021-08-10T17:22:31+05:30 In:

ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD ΔACD .Q.1(i)

  • 0
ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD ΔACD .Q.1(i)

Sir please help me to solve the ncert class 9th solution of chapter triangles.How I solve this question of exercise 7.3 question number 1(i). Find the simplest and easiest solution of this question , also give me the best solution of this question. ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD ΔACD .

best math ncert solutionexercise 7.3 ncertncert class 9th solutiontriangles ncert
Share

    1 Answer

    1. Guru

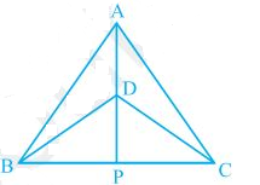
      In the above question, it is given that ΔABC and ΔDBC are two isosceles triangles.

      (i) ΔABD and ΔACD are similar by SSS congruency because:

      AD = AD (It is the common arm)

      AB = AC (Since ΔABC is isosceles)

      BD = CD (Since ΔDBC is isosceles)

      ∴ ΔABD ΔACD.

      • 0

    Related Questions

    Adv