important 5 mark board question
Speed of a boat in still water is 15 km/h. It goes 30 km upstream and returns back at the same point in 4 hours 30 minutes. Find the speed of the stream.
Share

Lost your password? Please enter your email address. You will receive a link and will create a new password via email.
Given: Speed of boat in still water = 15 km/hr.
Let the speed of stream = x km/hr.
Distance covered by boat in going upstream = Distance covered by boat in downstream = 30 km
Speed of boat upstream = Speed of boat in still water – Speed of stream = (15 – x) km/hr.
Speed of boat downstream = Speed of boat in still water + Speed of stream = (15 + x) km/hr.
Since, Distance = Speed × Time
⇒ Time = Distance / Speed
Time taken by boat upstream =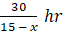
Time taken by boat downstream
Total time taken by the boat to go and come back to the same point = 4.5 hrs.
∴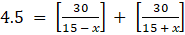
Multiply and divide both sides by (15 – x)(15 + x):
⇒ 4.5 (225 – x2) = 30(15 + x) + 30(15 – x)
⇒ 1012.5 – 4.5x2 = 450 + 30x + 450 – 30 x
⇒ 1012.5 – 4.5x2 = 900
⇒ 4.5x2 = 112.5
⇒ x2 = 25
⇒ x =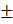 5
5
But speed can’t be negative, ∴ Speed of the stream = 5 km/hr.